Vera W. de Spinadel
Centre of Mathematics & Design
Faculty of Architecture, Design and Urban Planning
University of Buenos Aires
E-mail: vwinit@fadu.uba.ar
Internet: http://www.fadu.uba.ar/maydi/

Abstract: In this paper, we present the Metallic Means Family (MMF), being the most paramount of its members, the Golden Mean f and in the second place, the Silver Mean s _Ag. We call them a family because, aside from carrying the name of a metal – the Golden Mean, the Silver Mean, the Copper Mean, the Bronze Mean, the Nickel Mean – they enjoy common mathematical properties that confer them a fundamental importance in modern investigations. Among these applications, we have chosen the analysis of the forbidden symmetries in a quasicrystal.

AMS Subject Classification: Number theory

Keywords: generalized Fibonacci sequences, Pisot-Vijayaraghavan numbers, Metallic Means Family

1. FIBONACCI SEQUENCES

A secondary Fibonacci sequence is a sequence of integer numbers, where every number is the sum of the two previous ones. Beginning with F(0) = 1; F(1) = 1, we get

1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144,...

(1.1)

where F(n + 1) = F(n) + F(n - 1).

Secondary Fibonacci sequences may be generalized, originating the so called «generalized secondary Fibonacci sequence» GSFS,

a, b, pb + qa, p(pb + qa) + qb,...

that satisfies relations of the type

G(n+1) = p G(n) + q G(n - 1)

(1.2)

where p and q are natural numbers. From (1.2) we get

.

Taking limits in both members and assuming that exists and is equal to a real number x – as will be proved in next theorem --, we have or else x² — px — q = 0, which positive solution is . This means

=

(1.3)

Theorem 1.

Given a GSFS

a, b, pb + qa, p(pb + qa) + qb,...

such that G(n+1) = p G(n) + q G(n-1), with p,q natural numbers, then there exists = s and it is a real positive number.

Proof: to find an expression for the n-th term of the GSFS, let us write equation (1.2) in the form G(n+1) = p G(n) + q H(n), where H(n+1) = G(n). Introducing the matrices

.

this last equation can be put in matricial form. In fact, it is easy to prove that or else .

Let us assume G(0) = G(1) =1 for simplicity. If then and the problem is reduced to the finding of the n-th power of matrix A. The characteristic equation of A is

,

with the two eigenvalues . To diagonalize A and convert it in , we shall use the change of base matrix . The n-th power of A is calculated applying the similarity transformation . Finally we have

.

so that .

Replacing we get

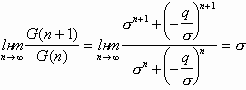
and the proof is complete for the sequence: 1, 1, p + q, p(p + q) + q,...

Note: If, instead of choosing G(0) = G(1) = 1 we begin with two arbitrary values a and b, it is easy to prove that the result is the same. Actually, given the GSFS: a, b, pb + qa, p(pb + qa) + qb,... we have to evaluate the ratio

.

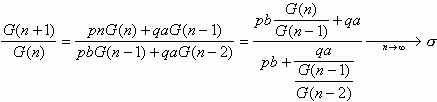2. THE FAMILY OF METALLIC MEANS

Let us consider a set of positive irrational numbers, obtained taking G(0) = G(1) = 1 in equation (1.3) and considering different values for the parameters p and q.

Definition: The Metallic Means Family (MMF) is the set of positive eigenvalues of the matricial equation

.

(2.1)

for different values of p and q (natural numbers).

All the members of this family are positive quadratic irrational numbers that are the positive solutions of quadratic equations of the type

.

(2.2)

Let us begin with . Then it is very easy to find the members of the MMF that satisfy this equation, expanding them in continued fractions. In fact, if p = q = 1, it results x² = x + 1, that can be written: Replacing iteratively the value of x of the second term, we have 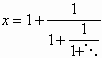, that is x = [1,1,1,...] = ,

a purely periodic continued fraction that defines the Golden Mean

.

Analogously, if p = 2 y q = 1 we obtain the Silver Mean

= [],

another purely periodic continued fraction.

If p = 3 and q = 1, we get the Bronze Mean

= = ,

For p = 4; q = 1, the Metallic Mean is , a striking result related to the continued fraction expansion of odd powers of the Golden Mean. Interesting to mention, odd powers as well as even powers of the Golden Mean have interesting and different continued fraction expansions in terms of Lucas numbers [1].

It is easy to verify that the following Metallic Means are

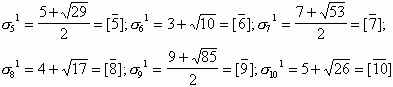
Obviously, all of them are of the form , a purely periodic continued fraction expansion. The slowest converging one of all them is the Golden Mean, since all its denominators are the smallest possible (ones). An elegant way of stating this result is

The Golden Mean f is the most irrational of all irrational numbers.

If, instead, we consider equation , we have for q = 1, again the Golden Mean. If p = 1 and q = 2, we obtain the Copper Mean , a periodic continued fraction. If p = 1 and q = 3, we get the Nickel Mean, Analogously

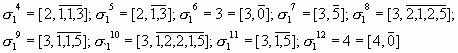
and all these members of the MMF are of the form . Furthermore, it is very easy to verify that in this set, the integer metallic means like appear in quite a regular way. The first for q = 2 (1+=2), the second for q = 6 (3+=6), the third for q = 12 (7+=12), the forth for q = 20 (13+=20), the fifth for q = 30 (21+=30), and so on, where .indicates the number of equations separating two consecutive integer solutions. The first digit of the Metallic Mean that is not equal to an integer is m = 2 for the first three; m = 3 for the second five; m = 4 for the third seven; m = 5 for the fourth nine, etc. The continued fraction expansions of the non integer Metallic Means are «palindromic», that is, the periods are symmetrical with respect to their centres, with the exception of the last digit of the period that is equal to 2m-1. There is no simple rule which predicts the length of the periods, some of them are very short like for example, the first after an integer solution has a period equal to 1: q = 3 (); q = 7 (); q = 13 (); q = 21 ();... and the last ones before an integer solution are of the form: q = 5 (); q = 11 (); q = 19 ();..., that is, they have periods of length 2. Other periods are extremely long and with the exception of the cases q = 3,7,13,21,31,... the remaining continued fraction expansions present «stable cycles» of different lengths.

3. PISOT AND SALEM NUMBERS

Let us consider the set U of real algebraic numbers greater than 1 whose remaining conjugates have modulus at most equal to 1. This set is divided into two disjoint sets, S and T. The set S of Pisot numbers was simultaneously and independently defined by Charles Pisot, 1910-1984, in his famous thesis published in 1938 [2] and Vijayaraghavan [3], [4]. Thus, S-numbers are called Pisot-Vijayaraghavan’s numbers or shortly PV numbers. The set of PV numbers is the set of real algebraic numbers q > 1 whose other conjugates have modulus strictly smaller than 1. The set T of Salem numbers (discovered by Raphael Salem, 1898-1963) is the set of real algebraic numbers t > 1 whose other conjugates have modulus at most equal to 1, one at least having a modulus equal to 1 [5], [6].

The sets S and T define a partition of U. All rational numbers greater than 1 belong to S. The quadratic numbers in S are zeros of second degree polynomials with integer coefficients. It is easy to prove that S is a closed set on the real line. The set of limit points of S is called the «derived set of S» and is denoted by S’ . Furthermore, if q is a PV number, then q ^{m О} S’ for every integer m і 2. This implies that all PV numbers of degree 2 О S’, being the smallest of them the Golden Mean, which is also the least element of S’.

It is evident that the PV numbers are a subset of the MMF, since the Metallic Means whose expansion in continued fractions is purely periodic are quadratic PV numbers because they are the positive solutions of quadratic equations of the form x² – px – 1 = 0, with p a natural number. In addition, the positive solutions of quadratic equations of the type x² – px + 1 = 0 where p і 3, are also PV numbers that admit purely periodic continued fraction expansions, where the condition that the terms of the continued fraction have to be positive, has been relaxed [7]. For example

.

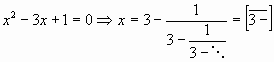On the contrary, there exist no examples of Salem numbers as simple as the ones given for PV numbers because it can be proved that there are no Salem numbers of degree less than 4. Notwithstanding, both sets of PV numbers and of Salem numbers have many important applications not only in the quasicrystalline context but also in the formal study of power series and harmonic analysis.

4. QUASICRYSTALS: FORBIDDEN SYMMETRIES

Quasicrystals gave diffraction patterns that show local n-fold rotational symmetry forbidden in Crystallography, that is n = 5, 8, 10, 12. This symmetry is combined with self-similarity. In fact, diffraction patterns of quasicrystalline structures exhibit sharp Bragg peaks in their diffraction pattern and noncrystallographic local n-fold symmetries of order n = 5, 8, 10, 12. In this context, quasilattices can be thought as mathematical discrete sets supporting Bragg peaks or atomic sites. They play the same role as lattices do for crystals.

Recently [8], [9], [10], [11], discrete sets of numbers, the b -integers Zb , have been proposed as numbering tools for coordinating quasicrystalline nodes in 1, 2 or 3 dimensions, and also the Bragg peaks in diffraction patterns [12], [13].

In the observed cases:

Golden Mean (penta- or decagonal quasilattices)

Silver Mean (octogonal quasilattices)

Subtle Mean (dodecagonal quasilattices) ¹

Interesting to mention, the continued fraction expansions of these irrational numbers are the following:

All of them have purely periodic continued fraction expansions.

The relevant scale factor b is a quadratic PV number[14], i.e. an algebraic integer b > 1 which is solution to equations of the type

()

such that all respective second roots b ’ (called Galois conjugates of b) have modulus strictly smaller than 1.

Think of the role played in lattice theory by the ring of integers Z and the planar rotational compatibility condition

Z

Lattices are generated through successive additions or substractions of a finite number of vectors. They are closed under the internal law

For lattice, lattice for all Z.

In quasilattices, the compatibility condition is replaced by

Zb

and obviously, Zb reduces to Z when is a natural number.

In terms of the members of the MMF, quadratic Pisot numbers have the following equivalences

The discovery of quasi-crystals with crystallographically forbidden symmetries is one of the most striking examples where a pure symmetry analysis determines mathematically forbidden symmetries appearing in a new solid state of matter.

From the technological point of view, quasicrystalline alloys, due to the intrincated interlocking connections among its components, are much harder than the customary crystals and can be advantageously used as substitutes of industrial diamonds In this context, quasilattices can be thought as mathematical discrete sets supporting Bragg peaks or atomic sites. They play the same role as lattices do for crystals.

Notice

•  ¹⁾  This number has been called the Subtle Mean by El Naschie [29], and besides appearing in quasiperiodic tiling and crystallography, it plays a significant role in the theory of Cantorian fractal-like micro-space-time. In addition, the Subtle Mean is involved in a fundamental way in various basic equations in knot theory, noncommutative geometry and four manifold theory [30].

References

[1] Vera W. de Spinadel, From the Golden Mean to Chaos, Nueva Librerнa (1998), second edition Nobuko (2004)..

[2] Charles Pisot, La rйpartition modulo 1 et les nombres algйbriques, Ann. Scu. Norm. Sup. Pisa, sйrie 127 (1938), 205-208.

[3] T. Vijayaraghavan, On the fractional part of the powers of a number, Proc. Camb. Phil. Soc. 37, (1941), 349-357.

[4] T. Vijayaraghavan, On the fractional part of the powers of a number, London Math. Soc. 17, (1942), 137-138.

[5] Raphaлl Salem, Power series with integral coeffcients, duke Math. Journ. 12, (1945), 153-173.

[6] Raphaлl Salem, Algebraic numbers and Fourier Analysis, Heath Math. Monographs, Boston, Mass., (1963).

[7] Vera W. de Spinadel, Half-regular continued fraction expansions and Design, Journal of Math. & Design, vol. 1, Number 1, March (2001), 67-71.

[8] D. Barache, B. Champagne and J. P. Gazeau, Pisot-Cyclotomic Quasilattices and their Symmetry Semi-groups, ed. J. Patera, Fields Institute Monograph Series, vol. 10, Amer. Math. Soc., (1998).

[9] J. P. Gazeau, Pisot-cyclotomic integers for Quasicrystals, The Mathematics of Aperiodic Long Range Order (ed. R. V. Moody) NATO-ASI Proceedings, Waterloo 1995, Kluwer Academic Publishers (1997).

[10] C. Burdik, C. Frougny, J. P. Gazeau. and R. Krejcar, Beta-Integers as natural counting systems for Quasicrystals, J. Phys. A: Math. Gen., 31, (1998), 6449-6472.

[11] J. P. Gazeau, Counting systems with irrational basis for Quasicrystals, editors: F. Axel, F. Dйnoyer, J. P. Gazeau. In From Quasicrystals to more Complex Systems, No. 13. Les Houches School Proceedings, Springer-Verlag, Heidelberg, Berlin, (2000).

[12] V. Elser, Indexing problems in Quasicrystal Diffraction, Phys. Rev. B32, (1985), 4892-4898.

[13] J. P. Gazeau and D. Lipinski, Quasicrystals and their Symmetries, Symmetries and structural properties of condensed matter, Zajaczkowo 1996. Ed. T. Lulek. World Scientific: Singapore, (1997).

[14] M. J. Bertin, A. Decomps-Guilloux, M.Grandet-Hugot, M. Pathiaux-Delefosse and J. P. Schreiber, Pisot and Salem numbers, Birkhдuser Verlag, 1992.

[15] M. S. El Naschie, Remarks to the PV Number chaos, solitons and Fractals, vol. 9, No. 8, (1998), 1445-1471.

[16] M. S. El Naschie, The Golden Mean in Quantum Geometry, Knot theory and related topics, Chaos, Solitons and Fractals, vol. 10, No. 8, (1999), 1303-1307.